Teaching positional chess to third and fourth graders, many of whom are beginners, resembles teaching William Faulkner's novels to college freshman. There is more in the lesson than the students have the time and ability to absorb. I have taught Faulkner's
Go Down, Moses (1942) as a unified novel in a college introductory literature class.* I teach positional chess concepts to third graders. I may be nuts.
Elementary Tactics
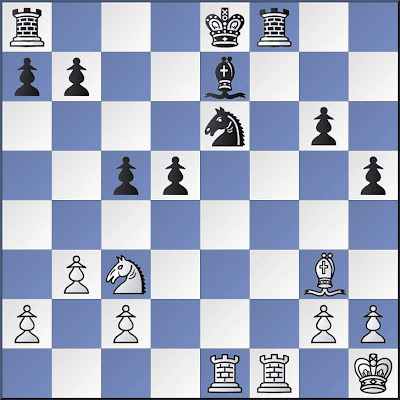
Beginners struggle to spot simple forks. Beginners capture pinned pieces, instead of piling on with a piece of less value. Indeed, even some of the successful third graders--measured in numbers of trophies earned since kindergarten--do not always handle an elementary pin in the correct manner. The first problem in my Knight Award Checkmates and Tactics set should be easy, but even players who have won trophies at the Washington State Elementary Chess Championship have erred while solving it.
White to move
I took this position from Barnes -- Mongredien 1862, but it appears in fourteen games in the ChessBase online database. The Black knight on c6 is pinned, so White easily wins material piling on the knight with 1.d5. Barnes made this move in the game, and then Mongredien replied 1...a6. While beginners often fail to see 1.d5, most of those with some experience find the correct move. Alas, after I ask about their reply to 1...a6, they fail. Even state trophy winners have told me they would capture the knight with the attacked bishop.
If a player cannot see to retreat the bishop, maintaining the pin and winning the knight for a pawn, he or she may not be ready for positional chess. Endless repetition of elementary tactics seems necessary first. My chess pupils may advance more rapidly if I induce each of their parents to purchase Bruce Pandolfini,
Beginning Chess (1993) and compel working through the 300 problems therein as a precondition to participation in tournaments.
In mid-October, I started creating worksheets with simple tactics and few pieces--problems inspired by the method in
Beginning Chess. Some groups required more than thirty minutes to solve the first six of these elementary problems (see "
Lesson of the Week" [16 October 2012]). The second and third weeks, the young players solved the problems more rapidly and with greater accuracy. Some beginners, however, continue to struggle.
This simple problem was one that many missed this week on their first try.
White to move
White has a simple tactic, but there is a more advanced element too. After the elementary fork, 1.Be5+ Qxe5 2.Rxe5 Kxe5, White has only one winning move, 3.Kg3. For the worksheet this week, students need only find the winning fork. This position may appear again later in pawn ending training.
Endgame Skills
The resulting king and pawn endgame requires understanding of opposition and outflanking. My chess students begin learning such lessons after earning the Knight Award. For the Bishop Award, a player must demonstrate understanding of Jeremy Silman's king vs. king exercise (see
How to Reassess Your Chess, 3rd ed. [1993], 3-8.), and two elementary king and pawn exercises. I play Black; the student must win both.
White to move
White to move
A student who has earned the Bishop Award has developed endgame skills well beyond the vast majority of scholastic chess players. In addition to understanding the rudiments of opposition and outflanking, he or she knows how to checkmate with two bishops, and has solved 64 chess problems in addition to those completed for the Knight Award. The Knight Award Checkmates and Tactics problem set consists of twelve problems. The Bishop Award Checkmates and Tactics problem set has 24. In addition, two sets of twenty problems must be solved from my "Checklist of Checkmates" workbook: Corridors and Diagonals.
Positional Chess
[E]ach chess move begins in the head. If you attach importance to playing a good game of chess, you must first of all learn how to think properly.
Alexander Bangiev, Squares Strategy CD
Even while observing a significant lack of skills in elementary tactics, I have been encouraging young players to consider the strategic elements. Until the late nineteenth century, the world's top chess players were masters of attack. None of them were particularly capable defenders. Modern players sometimes scoff at the alleged brilliance of some of these attacking games because even strong club players of today easily spot superior defensive moves. The attacking play of Adolf Anderssen, for example, appears rooted not so much in his tactical brilliance as in the wholly inadequate defensive play of his adversaries (see "
Understanding Mayet's Thinking" for an example).
As the nineteenth century gave way to the twentieth, positional play became the focus of top chess players. The elements of positional understanding were first articulated by Wilhelm Steinitz, followed by Siegbert Tarrasch, and others. Garry Kasparov asserts that Akiba Rubinstein was the "brightest" of the so-called Steinitz School that ushered in this era of positional play (
My Great Predecessors, vol. 1 [2003], 187). Since mid-September, my weekly lesson for young players has featured a position from the games of Rubinstein.
Over the course of the 2012-2013 school year, additional lessons will come from a small group of players from the beginnings of modern chess. In November and December, lessons will come from the games of Paul Morphy. There will be tactical positions in abundance, but I will labor to encourage students to understand the positional base from which these tactics became possible. Although considered an attacking player, study of Paul Morphy's play from the mid-nineteenth century reveals that he understood positional concepts. In January, we will turn our attention either to Emanuel Lasker or to Siegbert Tarrasch. The other will follow from mid-February through the end of March.
In
The Modern Chess Instructor (1889), Wilhelm Steinitz set out to articulate the "maxims of development" as the basis for emerging chess strategy (viii). He notes, as an example, how the French Defense deprives White of many sacrificial possibilities against the vulnerable f7 square. Consequently, when Black meets 1.e4 with 1...e6, White is forced to attend "to the balance of forces and position on all parts of the board, and the accumulation of small advantages if possible" (xxxii). Chess players since the time of Steinitz have been able to articulate that one side stands better in many positions even when material is equal and no immediate tactics are available. The position from "
Lesson of the Week" (3 October 2012) offers a useful illustration.
White to move
Most skilled chess players immediately notice that White has a lead in
development. That would have been a suitable answer when I posed the first of two questions that I introduced to young players the first week of October--the week that most of my school chess clubs began.
1. Which side is better?
2. What are the plans for both sides?
White is better due to a lead in development. However, most third graders cannot understand such abstractions. How does Steinitz define the term development? How does Tarrasch? How do other chess coaches working with young players communicate this abstract term so that it can be understood by those as young as six years old?
White's occupation of the center--four squares in the middle of the board--is much more concrete. Black is contesting two center squares. The one unoccupied center square, d5, is attacked by two Black pieces. Only the knight attacks e4, which is occupied by a White pawn. One White piece attacks (or defends) the e5 square occupied by White's knight. Two pieces protect the pawn on e4. White's king is the only piece that asserts control over d4, currently occupied by a White pawn. Counting makes it clear that White not only occupies, but more importantly
controls the center of the board.
Another concrete exercise that young players can easily learn is counting the total number of legal moves. It quickly becomes clear that White's pieces are more mobile than Black's. They have more squares to which they might move, even though most of the potential moves for both players would lose material.
Mobility and
Center Control are more concrete concepts than development.**
Lessons from Rubinstein
I began the school year with a lesson on truth in chess: "Every conceivable chess position has a truth that may be discovered" ("
Lesson of the Week" [18 September 2012]). In the illustrative position, Rubinstein capitalized on an error by his opponent. As a consequence, he reached a superior endgame. He had a material advantage, but he traded the material advantage for clear positional superiority. In that game, positional superiority was manifest in mobile passed pawns. One of these was sacrificed to promote the other. His opponent quickly removed the new queen from the board, but at the cost of a rook. This ending was the lesson for the
last week of September.
My two positional questions were introduced the first week of October, and then reinforced the second week.
That lesson came from a game that Rubinstein did not win. Instead, he secured a draw because his opponent erred in a critical endgame position. In mid-October, Students faced
the third key position from the games of Rubinstein in which they were asked to assess who stands better. In that position, from Nimzovich -- Rubinstein 1907, Black's control of the open file proves a contributing factor to greater piece
mobility. Even more telling, however, is that White's pieces seem to get in one another's way, while Black's
Piece Coordination facilitates an attack that wins material.
Even as I continued with these positional lessons from Rubinstein, however, attention in my after school chess clubs shifted towards elementary tactics. The young players did not get much of a chance to express their views on the position from Nimzovich -- Rubinstein because they were busy solving elementary problems. Knowing the problems would be too easy for some players, however, I was armed with photocopies of three different worksheets as the students entered the room. The newest beginners were given the six problems on the Elementary Tactics I worksheet (see "
Lesson of the Week" [16 October 2012]). Beginners who had been in last year's clubs were handed the Pawn Award Checkmates and Tactics worksheet (six one-move checkmates). More advanced students were given the Knight Award Checkmates and Tactics worksheet. My top third grade players may have brought home a team trophy from state last year, but none of them have completed this worksheet and earned the Knight Award (there are additional requirements).
During the last two weeks of October (and carrying over to 1 November for the Thursday afternoon club), the lessons from Rubinstein received greater attention. Even though I continued to give players elementary worksheets as they entered the room, these were completed more rapidly. I continue to insist that players begin their offer of solutions to the problem on the demo board with a clear assessment of "who stands better?" No player may blurt out a possible move before offering this assessment. Even so, there are times when positional analysis must be subordinate to tactical calculation. Recognizing such moments becomes possible when tactical training develops pattern recognition. Also, the positional element of
Vulnerability comes into consideration.
Forks were prominent both in the Elementary Tactics II problems presented to the young players at the start of club the week before Halloween, and in the position from Rubinstein -- Hirschbein 1927. Rubinstein's combination in that game is his only representation in the
Anthology of Chess Combinations (1995) ("
Lesson of the Week" [24 October 2012]). Many students saw the fork on f6, but fewer were able to assess why it does not work, and then find the tactic--removing the guard--that renders the fork a viable threat. One first grader, however, after being the first in his club to solve the six easy problems, suggested Rxd7! I was less strenuous in stressing positional evaluation as the prerequisite to suggesting this move, but did walk him through some positional analysis before acknowledging that he found Rubinstein's move.
In the
final weekly lesson from Rubinstein's games, he is down two pawns and his queen is attacked. Many players were quick to observe the
vulnerability of the White queen. Fewer noticed that Rubinstein was down two pawns. I should be pleased that the young players are not so materialistic as to count all the pieces before seeking to rescue the queen. But, they need to see the corresponding vulnerability of the Black king. The White queen is safe so long as the Black king is in check.
Notes
*
Go Down, Moses was first published as
Go Down, Moses and Other Stories. Some readers consider it a collection of seven works of short fiction, somewhat related to one another. Others consider it a unified whole. I side with the latter group.
**My debt to Dan Heisman should be clear to well-read chess players. For many years, I have used
Elements of Positional Evaluation, Rev. ed. (1999) as my key text for articulating positional concepts. More recently, I have been studying
Elements of Positional Evaluation, 4th ed. (2010). Heisman's work deserves the attention of all serious chess players and teachers. Heisman refers to
development as a pseudo-element. The Elements, he argues, are Mobility, Flexibility, Vulnerability, Center Control, Piece Coordination, Time, and Speed. He might note that even Steinitz, in the effort to define development and the relative value of the pieces, employs mobility:
The superiority of the Bishop over the Knight is also shown by the fact that the former when placed on any square of the board will command at least 7 squares of one or more clear diagonals. In the middle of the board at K4, K5, Q4 or Q5, he will command 13 squares. On the other hand, the action of the Knight may be reduced to the command of no more than two squares, if he be placed into any of the four corners of the board, and the maximum of squares which he can command is eight. (The Modern Chess Instructor, xxxviii)
The elements put forth by Heisman are already present in the writings of Steinitz, Tarrasch, Lasker, and others of their era.